Wed June 19

Fix a geometry. Define a tile as a convex figure bounded by geodesic arcs.
Problem: Make a list of tiles where each angle is either pi/n or 2pi/n with symmetry.


HOMEWORK:
1. Anand will work out the problem for plane geometry (that is, in R^2).

2. Jingyu will work out the problem for spherical geometry (that is, in the sphere). (The answer is an interesting finite list and a not-so-interesting infinite list)


3. Matt may work out this problem  for hyperbolic geometry LATER

4. Robert: Get a tiling of the plane by hexangons. Consider the group of symmetries with no fixed points. Determine which surface is obtained.

5. Google Russell's paradox. (Wikipedia will be a good source). Read the contents. It will be discussed next week.

6 Jingyu will try to formalize the argument proving that in a simply connected space a tiling will "fit".

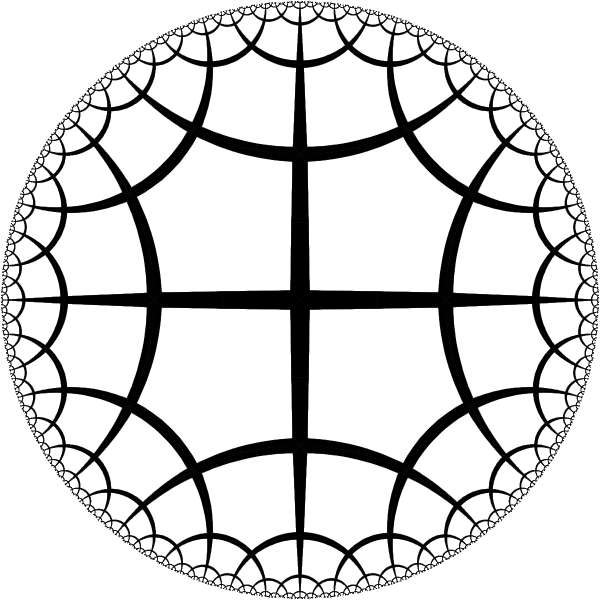
The picture below is a tiling of the Poincare disk by pentagons with right angles.